Dynamical High Speed Compaction of Metal Powder: University of the Ryukyus Repository

(1)

Title

Dynamical High Speed Compaction of Metal Powder

Author(s)

Miyagi, Kiyohiro

Citation

琉球大学理工学部紀要. 工学篇 = Bulletin of Science &

Engineering Division, University of the Ryukyus.

Engineering(10): 15-26

Issue Date

1975-09-01

URL

http://hdl.handle.net/20.500.12000/26600

(2)

D

y

n

a

m

i

c

a

l

H

i

g

h

Speed C

o

m

p

a

c

t

i

o

n

o

f

M

e

t

a

l

Powder

Kiyohiro MIYAGI

*

Summary

In this paper Experimented on the dynamical compaction of metal powder and compared the results with theoretical calculation.

U sing a rubber propelled press that passed through the press guide and directly impacted on the face of the powder in a die. The velocity of the press was controlled by changing the number of rubber bands. Using an acryl plate of appropriate strength

，

an author observed the progression of both the press and the shock

wave that were produced.

On the theoretical calculations an author overlooked the elastic recovery factor; the effect of the kinetic state of powder and the friction between the powder and die wallj the e任ect of the air that was mixed with the powder particle's.

On both experimental and theoretical calculation the final density and shock wave velocity were examined.

1 . Introduction

15

In the production methods of powder， there are the statical and the dynamical. The dynamical method produces a higher pressure than the statical method and moreover

，

there is the advantage of homogenized produced powder since comparati-vely there is less friction

，

which occurs due to greater longitudinal pressure than transverse

，

between the powder and die wall.

(1)

There are a great number of research， especially in production methods;"struc -ture:2

) and soliditydistribution~3) being carried out in this field， but only experimen-tally

，

and seemingly research into the process of compression is non-existent.

In this paper

，

we will examine both theoretically and experimentally the compac-tion process of powder by the insertion of powder into a die. In this examination

，

we will take a uniform quality unit of powder which is composed of a mixture of solid particles and air.

Generally in a graph it is known that the pressure and density curve is an upward one， consequently， phenomenon of experiment can assume that shock waves are created when there is an impact on the powder. The treatment of shock waves through a medium can be determined by the relationship of conservation laws of

Received: April30， 1975

(3)

16 MIYAGI: Dynamical High Speed Compaction of Metal Powder mass. momentum and energy and the state of the medium.

Determining the standard as the statical pressure and density condition of powder

，

on the calculation

，

theory treat the problem of the dynamical method using this standard and the conservation laws of mass and momentum. Moreover

，

in order to treat the problem of reflected waves more easily

，

we will overlook the elastic recovery factor. The experimental aspect will be observed on this paper by the use of a high-speed camera

，

which will follow the shock waves through the impacted

powder， and will be later compared with theoretical calculations.

2.

Basic Equation

2.1When the die is infinitely long.

Taking the [x J axis as the longitudinal direction of powder in a die

，

and a small element in the [x J axis between point

[

1

J and [nJ of length (dx) and let the cross sectional area of inside of die [AJ， we will consider that when mass [MJ impacts on the initial surface of the powder

，

then x = 0 and the velocity will be [ vo].

Taking time as [t.J

，

at some time the shock waves will reach a point [IJ

，

and later at time (dt) the shock waves will reach point [n J.

In the area where the shock wave face has already passed (back) the pressure is ( p) density is (ρ)， and particle velocity is (v) ; and at the area in front of the shock wave face the pressure is(長)， density is (ρ) and particle velocity is(骨).

When the velocity of the shock wave is (c)， then from application of the theory Low of conservation of mass

，

may be writen equation (1 ).

β ( c -" ) =ρ(c -，，) ( 1 )

From the relationship of momentum and impulse

，

we have equation (2).

p-t=β(c -ii) (" -ii ) ( 2 ) At the unimpacted state of the powder pressure and particle velcity of this powder is 0， (長=骨=0 ); then density (t) equals (ρ。)，(t

=

ρ。).

from equations (1) and (2) substituting becomes. ρo c -ρ( c - v)

p=ρo c v

( 3 ) (4 ) Overlooking the elastic recorvery factor， the area that is being pushed between the face of the impacting mass [MJ and the face of the shock wave will have the same velocity as the velocity of the impacting mass.

When the impacting mass is [MJ， and the area that being pushed is (m)， the small area between a point

[

1

J and a point [n J will be (dm).

Velocity of press is decrease by resistance of powder

，

in the area where passed shock wave

，

in consequence of elapsed time pressure is gradualy decrease

，

when

(4)

Bull.Science& Engineering Div.， Univ. of the Ryukyus(Engineeringl 17

overlooked the elastic recovery factor， in the removal process ofpressure the density remain unchanged， therefore

，

insuch area

，

the density of each point depend

on pressure by just after shock wave face passed， and keep up unified density， After press impacted powder

，

the element between shock wave face and press

willmove with same velocityas rigid body.

Consider the press and rigid body of powder

，

from the low of conservation of momentum

，

we arrive at equation(5).

-A

T

dt = (M + m + dm) (v十dv)- (M + m) v - dm骨

(M+m) dv+dr.:.(v -量) ( 5 )

Taking a facialcross-section ofthe powder as[A ] equation(5) becomes dm=ρA (c -v) dt (M + m ) 11+ρA (c-v) (v一面)+ At = 0 d t From equation(1 ，) (2)， (5)' we obtain

(

M

十m)

告

+ A

P

=

0

( 5 )， (6 ) We us e condition

T

= 云

o

from equation(5) we arrive at equation(7). d 1 (M+m) v I = 0 where (M + m) v = constant

From m = 0 and v = Vo at the just after impacted

( 7 )

Mv.

可=三一一一ーム一一

M+m

( 8 )

Consider shock wave just after passed optional point(x) at the longitudinal where unchanged powder particles.

m=tAx -ρ

。

Ax

(

9 )

Therefore particles velocity is

M v

，

v =

M+ρ

。

Ax (10)

In the statical method experiment， the Kawakita equation can be used in the relationship of pressure and density.

( p) and (ρ) will be used to describe pressure and densityina die. (a) and (b)

is the co旬e任icientof powder.

From relationofpressur (p) and (ρ) give ρ =

1+b

p -l+(b-ab)p 内 ) ' E A - - A (

(5)

18 MIYAGI : Dynamical High Speed Compaction of Metal Powder

From equations (3) and (4)， we eliminate shock wave veloclty (c) and arrive at equation(12).

v2

p

= 1

/

瓦コ

ρ

7

(12) From equation(11)and (12)we eliminate density (ρ)

a b p2 - bρ

。

v2P一ρ

。

v2=0 (12' ) From here we set. α=ab

，

β=bρ。v2

，

r

=ρ

。

v2

β +

ぷ写瓦

γ

2 α

(13)

From equation(4) becomes equation(14)and (15)

c -

士=去

(v

+

広

三

(14)

t

=

f

Ldx (1

5 )

Further from equation(14) we obtain equation (16)

bMv。 [EE-E里 +1…~/

2 A L v Vo ~ I

内

一

府

内市

(16) However K

=必チ

From equations (10)(11)and (13) we will know the density of

v opu

the area between the face of the impacting mass and that of the shock wave. 2.2 When the die is finite

In a finite die， the impacted shock wave will be reflected by the fixed end and will reflect back to the impacting mass [M ] .

Overlooking the elastic recovery factor， the pressure， the density and the par-ticle velocity in the area in front of the shock wave face ane (ji)， (p) and (苛) respectively， and in the area in the back will be (p)， (ρ) and (v).

The equation of continum and momentum are the same as equations (1) and ( 2 ). ρ(c-v)=p(c一言) p-ji = p (c 量)(v一奇) (17) (18) In this case， since v = 0， we obtain

(6)

Bull.Science & Engineering Div.， Univ. of the Ryukyus(Engineeringl 19 ρ ( c -v)

=

jJc (19) p -t = t c v (20) From equation (19)， (20) and next equation gives. 1十b

T

- l+(b-ab)t ρ

。

(21) β +

原芋

4ai 2& (22) However. a = b (ρ

一

。

ρ+aρ) β= (ρ一ρ。)

(

1

十bt)+ bρ ( a p +ρ。v2₎ ₍₂₃₎ γ=ρ

。

pρp+ρ

。

ρv2

Moreover after shock wave refrected from press

，

when shock wave progress-ing to fixed end. at the [x] axis front of shock wave face

，

particle velocity云always

equal zero. Identicaly we have. ρ (c-v)=jJ c p-p ρ c v In case equation(1

3 )

ofα，β，γbecomes. α= b (P. -

P

+

aρ)

i

β = (ρ-ρ

。

)(

l

-

ht

)

+bt

(

at

十ρ

。

v

2₎ ₍ γ=ρ

，

P

-

t

5 T

+

ρ

，

P

v2 ) (24) (25) (26)

From relation of momentum of back of shock wave face

，

we get existence (5)'

and (6) at the reflected wave.

when refJected wave at the fixed end go back to press， mass of powder (m) abtain.

m= m.+ A

J

I

ρc dt (i=1， 3，5， ...)

(

2

7 )

When shock wave progressing to the fixed end， mass of powder (m) at the front of shock wave face is

m=AJtc dt ( j =0， 2， 4， 6，・・・・・ ) (28)

discrived before uniform pressure

T

anb density were known. However from (19) (20)， (5) ， (13)， (28) we decideρc， v， p， m.

(7)

20 MIYAGI: Dynamical High Speed CompactionofMetal Powder

3. Experiment

3.1 Experimental material

A material of powder were used 200 mech of copper powder as the metal powder to be impacted upon. Since vertical position the force of gravity wil not allow for a homogenized state of powder in terms of density， we packed the copper powder as best as we can into the die horizontally.

3.2 Experimental Equipment and method for Dynamical impact

Equipment of Experiment will used the die is the vertical position as indicated Figure 1 in consideration for better efficiency of the press durring its passage

1 . pressguide 2. back plate 3. die of high speed pressure method 4. acryl plate 5. thick acryl plate 6. holder of theacrylplate Fig. 1 Aapparatus for the measurement of shock waves usinga high speed camera in thedynamicalhighspeedpressure method

to the powder that is to be impacted upon. In order to observe the change of the powder a high-speed camera as it is being impacted， Die of dynamical pressure method were made one of the four walls transparent by used of acryl wall and

which have appropriate strength.

Photograph 1 and cross-section of the equipment used as shown in Fiure 2 respectively.

Inorder to create on accelerated passage of the press

，

it was convienient to

use tension from a rubber powerd source， which has the advantage of controlof

(8)

Bull. Science & Engineering Div.， Univ. of the Ryukyus (Engineeringl 21

Photo. 1 Equipments of dynamical high speed compaction experiment.

。。

。

。。

~IIII ~

I

II~

0

1

1 ~

1

1 ~

0

1

。

1 . back wall _{4. t}_h_i_n_acryl_p_l_a_t_e 2. supports 5. thickacryl plate

3. lowe土press 6. pressure plate Fig. 2 Die ofdynamical high speed compaction.

(9)

MIYAGI : Dynamical High Speed Compaction of Metal Powder

The measurement of the die chamb町・ as a rectanguler cross-section is 11.65

x

36

(mm)， and this chamber when filled with 75 grams of copper powder will be 12cm in depth and 6 cm in depth when filled with 3

7

grams. as indicated by Table

1 ，

used form kinds of press in this impact experiments.

we 22 Form kinds of press mass [M] kg. s2/m velocity v

，

/s 1 0.0156 46 2 0.0312 43 3 0.0468 41 4 0.0624 39 I Table

passing through the press guide in its passage towards impact on the powder

，

we used a photo transtor in the measurement of the velocity of the press as shown

Figure 3. In n A ν . 1 t F a ﹃も︾ e 3 F 目A . e 3 . t 3 d 恥 U_い n v σ e n e sι ﹄ e o a F I p o

一

L1 L2 Lj， L2・lamp Rl>R2 : resistor P l'P 2 : photo transistor・

一

_一

一

_一

start signal stop signal universalcounter A device to measure thevelocity ofthe press

In the period just prior to contact with the medium sarface

，

the press will activate a photo-transistor trigger

，

which will in turn activate a strobo Iight which durring its period of activation， will aHow the high-speed camera to record the events， The high-speed camera photo graphed at 40，000 frames per second. we list the parts

(10)

Bull.Science& EngineeringDiv.. Univ. ofthe Ryukyus(Engineering) 23

oftheequipment used in theexpriment as follows. the numbers are indicated photo 1. 1. press guide

2. miror for highspeed camera

3. strobo flasher 4. rubbers

5. unioversal counter

6. network forstrobo flashand u'niversalcounter

7. high speed camera

8. die of dynamical high speed compaction

1 . universal testing machine 2. upper press 3.die of statical pressure method 4. dialgauge 5.copperpowder 6. lower press 7. deskof universaltesting machine Fig. 4 Measurement of the shrinkage ofpowder at the staticalpressure method. 3.3 Statical compaction method

In the statical compaction methods. we used

1

0

grams of copper powder in a

statical pressure die， pressed the powder by a Universal testing machine and measured the shrinkage of powder by two dialgauge indicated as Figure 4

4. Results of Experiment and the Theoretical Calculation.

Results of statical compaction method experiment is shown by Figure 5

Vertical axis represents pressure that is divided by powder strain and horizontal

axis represents pressure from this Figure 5 using theKawakita equation we have

r .oefficients a and b. aニ

0 .

8

，

b

=

0 .

8

0

6

X

1

0 -

6 p/ε 6DOO 5000~ 品

。

0 0 4 1 K G 、凶ぷ 3000 2000 1000 10凹 2000 3000 4000 Pressur1:!/kgi佃川 Fig. 5 Resultsof statical compactionmethod

(11)

24 MIYAGI : Dynamical High Speed Compaction ofMetal Powder

However a is the reciprocal of the tangent of a straight line.， and l/ab becomes the intersect.

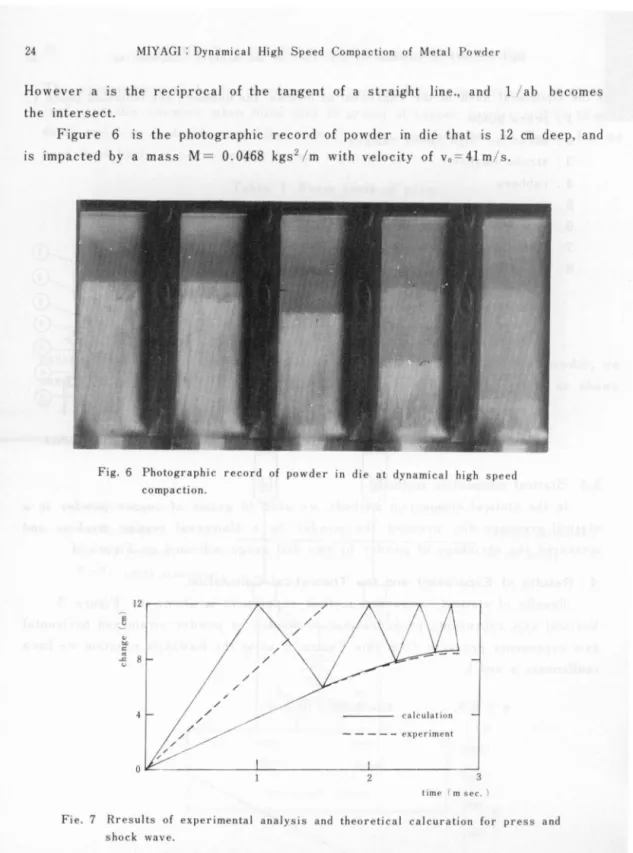
Figure 6 is the photographic record of powder in die that is12 cm deep， and

is impacted by a mass M

=

0.0468 kgs2/m with velocity of v.= 41 m/s.

Fig.6 Photographic record ofpowder in dieatdynamical highspeed

compaction. 12 n 6 ω 回目伺﹄ ω / / / {_E / / / / / ー一一一一一一一-calculation ー一一一-e:xperiment 3 time(m sec.)

Fie. 7 Rresults of experimental analysis and theoreticalcalcurationfor pressand shock wave.

(12)

Bull.Science & Engineering Div.， Univ. of the Ryukyus (Engineeringl 25 800 2.8msec トー 2.2m sec トー l.Om sec. E 、 ω J:f600 凶 W C U 司 400 200 6 12 length of pl)wder (cm) Fig.8 Time lapseandchange of density during time progression.

From this photograph we could realize the shock waves that is created. Figure 7 indicates results of experimental and theoretical calculations; with the experimental calculation deriving from the meassurement of the progression of both shock wave and the press. Fig.8 indicates results of theoretical calculation at time lapse and change of density during time p-rogression.

5. Conclusion

When the copper powder is dynamically compact in the finete die.

1. we theorized a fundamental equation， and from this calculated and experimented as described previously. In finite condition of die theoretical calculation was derived by numerical deference equation method.

2. In the Dynamical Compaction method， the compaction process was recorded by a high-speed camera， and the results recorded showed that shock waves were produced.

3. In comparing the experimental results with that of theoretical calculations

，

the final density of the powder coincide， but there is a discrepancy in relation to

shock waves.

4. The reasons for the discrepancy in relation shock waves are

a) Fundamental equation

，

we used the statical condition of powder as the standard. (Ka wakita equation)

(13)

26 MIYAGI : Dynamical High Speed Compaction of Metal Powder

b) we overlooked both the effects of the state of powder and the friction between the powder and die wall.

c) in the experiment we overlooked the effect of air that was in the die wit h the powder particles.

References

1 ) Nomura Kogyo kayaku Kyokai-shi 22 -6(1961) 321・329

Seimitu Kikai 31・7(1965) 617・623

2) Yasuyuki Kimura 3) Moriya Oyane 4) Tamio Kawakita

Huntai oyobi Hunmatu-Yakin 15-5(1968) 245・253