On Beam
Splittings
and Mathematical
Construction
of Quantum Logical Gate
Wolfgang $\mathrm{F}\mathrm{r}\mathrm{e}\mathrm{u}\mathrm{d}\mathrm{e}\mathrm{n}\mathrm{b}\mathrm{e}\mathrm{r}\mathrm{g}^{\uparrow}$
,
Masanori Ohya* and Noboru Watanabe**Department of Information Sciences,
Science University of Tokyo
Noda City, Chiba 278-8510, Japan
\dagger Brandenburgische Technische Universit\"at Cottbus,
Fakult\"at 1, Institut f\"ur Mathematik, PF 101344, D-03013 Cottbus,
Germany
Abstracts
In usual computer, there exists an upper bound of computational speed because of irreversibility of logical gate. In order to avoid this demerit, Fredkin
and Toffoli [3] proposed
a
conservative logicalgate. Basedon
theirwork,Milburn[4] constructed a physical model of reversible quantum logical gate with beam
splittings and a Kerr medium. This model is called FTM (Fredkin -Toffoli
-Milburn gate) in this paper.This FTM gate was described by the quantum
channel and the efficiency of information transmission of the FTM gate was
discussed in [10]. FTM gate is using a photon number state as
an
input statefor controlgate. The photon numberstate might bedifficulttorealizephysically.
In this paper, we introduced a new device on symmetric Fock space in order to
avoid this difficulty.
In Section 1, we briefly review quantum channels and beam splittings. In
Section 2, we explain the quantum channel for FTM gate In Section 3, we
intruduced a new device on symmetric Fock space and discuss the truth table
for
our
gate.1.
Quantum
channels
Let $(\mathrm{B}(\mathcal{H}_{1}), \mathfrak{S}(\mathcal{H}_{1}))\mathrm{a}\mathrm{n}\mathrm{d}(\mathrm{B}(\mathcal{H}_{2}), \mathfrak{S}(\mathcal{H}_{2}))$ be input and output systems, respec-tively, where $\mathrm{B}(\mathcal{H}_{k})$ is the set of all bounded linear operators on a separable
Hilbert space $\mathcal{H}_{k}$ and $\mathfrak{S}(\mathcal{H}_{k})$ is the set of all density operators on $\mathcal{H}_{k}(k=1,2)$.
(1) $\Lambda^{*}$ is
linear
if$\Lambda^{*}(\lambda\rho_{1}+(1-\lambda)\rho_{2})=\lambda\Lambda^{*}(\rho_{1})+(1-\lambda)\Lambda^{*}(\rho_{2})$ holds for any$\rho_{1},$$\rho_{2}\in \mathrm{e}(\mathcal{H}_{1})$
and
any $\lambda\in[0,1]$.
.(2) $\Lambda^{*}$ is completely positive $(\mathrm{C}.\mathrm{P}.)$ if $\Lambda^{*}$ is linear and its dual $\Lambda$ : $\mathrm{B}(\mathcal{H}_{2})arrow$ $\mathrm{B}(\mathcal{H}_{1})$ satisfies
$\sum_{i,j=1}^{n}A_{i}^{*}\Lambda(\overline{A}_{i}^{*}\overline{A}_{j})A_{j}\geq 0$
for any $n\in \mathrm{N}$, any $\{\overline{A}_{i}\}\subset \mathrm{B}(\mathcal{H}_{2})$ and any $\{A_{i}\}\subset \mathrm{B}(\mathcal{H}_{1})$, where the dual
map $\Lambda$ of$\Lambda^{*}$ is defined by
$tr\Lambda^{*}(\rho)B=tr\rho\Lambda(B)$, $\forall\rho\in \mathfrak{S}(\mathcal{H}_{1})$, $\forall B\in \mathrm{B}(\mathcal{H}_{2})$
.
(1.1)Almost all physical transformation can be described by the CP channel [5],
[7], [8]
Let $\mathcal{K}_{1}$ and $\mathcal{K}_{2}$ be two Hilbert spaces expressing noise and loss systems,
respectively. Quantum communication process including the influence of noise and
ioss
is denoted by the following scheme[6.]:
Let $\rho$ be an input state in$\mathfrak{S}(\mathcal{H}_{1}),$ $\xi$ be a noise state in 5 $(\mathcal{K}_{1})$
.
$\mathfrak{S}(\mathcal{H}_{1})\ni\rho$
$\frac{\xi\in \mathfrak{S}(\mathcal{K}_{1})\downarrow}{\downarrow}$
$\rho\in\Lambda^{*}\rho\in \mathfrak{S}(\mathcal{H}_{2})$
Loss
$\mathfrak{S}(\mathcal{H}_{1})$ $arrow\Lambda^{*}$ $\mathfrak{S}(\mathcal{H}_{2})$
$\gamma^{*}\downarrow$ $\uparrow a^{*}$
$\mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{K}_{1})$
$rightarrow\Pi^{*}$
$\mathfrak{S}(\mathcal{H}_{2}\otimes \mathcal{K}_{2})$
The above maps $\gamma^{*},$ $a^{*}$ are given as
$\gamma^{*}(\rho)$ $=$ $\rho\otimes\xi$, $\rho\in \mathfrak{S}(\mathcal{H}_{1})$, (1.2)
$a^{*}(\sigma)$ $=$ $tr_{\mathcal{K}_{2}}\sigma$, $\sigma\in \mathfrak{S}(\mathcal{H}_{2}\otimes \mathcal{K}_{2})$ , (1.3) The map $\Pi^{*}$ is a channel from $\mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{K}_{1})\mathrm{t}\mathrm{o}\mathfrak{S}(\mathcal{H}_{2}\otimes \mathcal{K}_{2})$determined by physical
properties of the device transmitting information. Hence the channel for the above process is given as
for any $\rho\in \mathrm{e}(\mathcal{H}_{1})$. Based on this scheme, the attenuation channel and the noisy
quantum channel
are
constructedas
follows:(1) Attenuation channel $\Lambda_{0}^{*}$
was
formulated suchas
$\Lambda_{0}^{*}(\rho)$ $\equiv tr_{\mathcal{K}_{2}}\Pi_{0}^{*}(\rho\otimes\xi_{0})$
$=$ $tr_{\mathcal{K}_{2}}V_{0}(\rho\otimes|0\rangle\langle 0|)V_{0}^{*}$, (1.5)
where $\xi_{0}=|0\rangle\langle$$0|$ is the
vacuum
state in $\mathfrak{S}(\mathcal{K}_{1}),$ $V_{0}$ isa
mappingfrom $\mathcal{H}_{1}\otimes \mathcal{K}_{1}$ to $\mathcal{H}_{2}\otimes \mathcal{K}_{2}$ given by
$V_{0}(|n_{1}\rangle\otimes|0\rangle)$ $=$ $\sum_{j}^{n_{1}}C_{j}^{n_{1}}|j\rangle\otimes|n_{1}-j\rangle$, (1.6)
$C_{j}^{n_{1}}$ $=$ $\sqrt{\frac{n_{1}!}{j!(n_{1}-j)!}}\alpha^{j}(-\overline{\beta})^{n_{1}-j}$ (1.7) $|n_{1}\rangle$ is the
$n_{1}$ photon number state vector in $\mathcal{H}_{1}$ and $\alpha$ and $\beta$
are
complex numbers satisfying $|\alpha|^{2}+|\beta|^{2}=1$. In particular, for the
coherent input state $\rho=|\theta\rangle$ $\langle\theta|\otimes|0\rangle\langle 0|\in \mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{K}_{1})$ ,
we
obtain the output state of$\Pi_{0}^{*}$ by$\Pi_{0}^{*}(|\theta\rangle\langle\theta|\otimes|0\rangle\langle 0|)=|\alpha\theta\rangle\langle\alpha\theta|\otimes|-\overline{\beta}\theta\rangle\langle-\overline{\beta}\theta|$ .
$\vdash^{\rho_{\theta}}\mathrm{X}^{-\beta\theta}1$
F.ig
1.1 Beam Splitting $\pi_{0^{*}}$Lifting $\mathcal{E}_{0}^{*}$ from $\mathfrak{S}(\mathcal{H})$ to $\mathfrak{S}(\mathcal{H}\otimes \mathcal{K})$ in the sense of Accardi and
Ohya [1] is denoted by
$\mathcal{E}_{0}^{*}$ (or $\Pi_{0}^{*}$)
is13
called a beam splitting. Basedon
liftings, thebeam splitting was studied by Accardi - Ohya and Fichtner
-Freudenberg- Libsher [2].
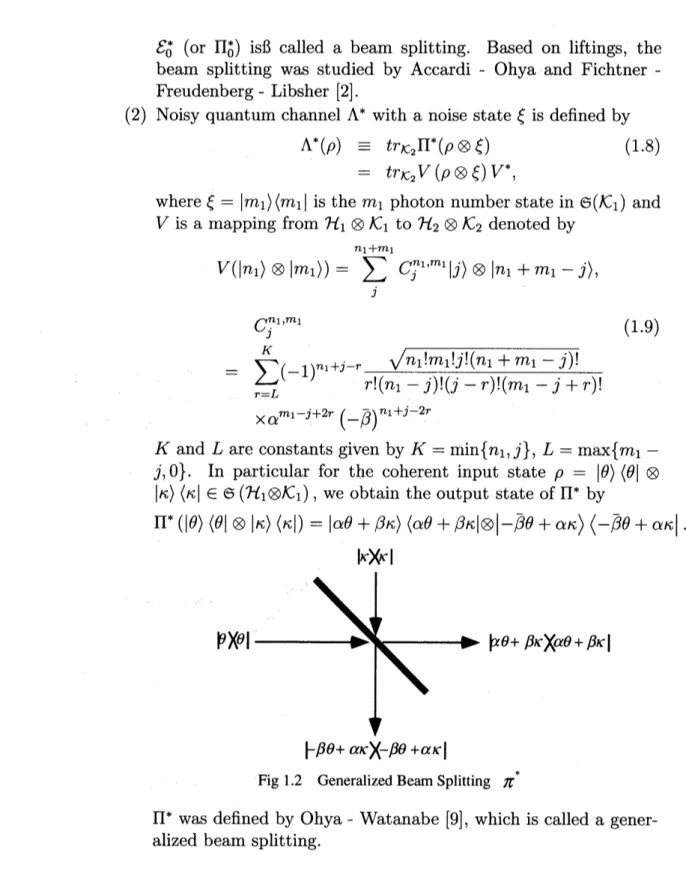
(2) Noisy quantum channel $\Lambda^{*}$ with a noise state $\xi$ is defined by
$\Lambda^{*}(\rho)$ $\equiv$ $tr_{\mathcal{K}_{2}}\Pi^{*}(\rho\otimes\xi)$ (1.8) $=$ $tr_{\mathcal{K}_{2}}V(\rho\otimes\xi)V^{*}$,
where $\xi=|m_{1}\rangle\langle$ $m_{1}|$ is the $m_{1}$ photon number state in $\mathfrak{S}(\mathcal{K}_{1})$ and $V$ is a mapping from $\mathcal{H}_{1}\otimes \mathcal{K}_{1}$ to $\mathcal{H}_{2}\otimes \mathcal{K}_{2}$ denoted by
$V(|n_{1} \rangle\otimes|m_{1}\rangle)=\sum_{j}^{n_{1}+m_{1}}C_{j}^{n_{1},m_{1}}|j\rangle\otimes|n_{1}+m_{1}-j\rangle$,
$C_{j}^{n_{1},m_{1}}$ (1.9)
$=$ $\sum_{r=L}^{K}(-1)^{n_{1}+j-r}\frac{\sqrt{n_{1}!m_{1}!j!(n_{1}+m_{1}-j)!}}{r!(n_{1}-j)!(j-r)!(m_{1}-j+r)!}$
$\mathrm{x}\alpha^{m_{1}-j+2r}(-\overline{\beta})^{n_{1}+j-2r}$
$K$ and $L$ are constants given by $K= \min\{n_{1}, j\},$ $L= \max\{m_{1}-$
$j,$$0\}$. In particular for the coherent input state $\rho=|\theta\rangle$ $\langle\theta|\otimes$ $|\kappa\rangle\langle\kappa|\in \mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{K}_{1})$, we obtain the output state of$\Pi^{*}$ by
$\Pi^{*}(|\theta\rangle\langle\theta|\otimes|\kappa\rangle\langle\kappa|)=|\alpha\theta+\beta\kappa\rangle\langle\alpha\theta+\beta\kappa|\otimes|-\overline{\beta}\theta+\alpha\kappa\rangle\langle-\overline{\beta}\theta+\alpha\kappa|$.
Fig 1.2 Generalized BeamSplitting $\pi^{\tau}$
$\Pi^{*}$ was defined by Ohya-Watanabe [9], which is called
a
gener-alized beam splitting.2.
Quantum
channel for
$\mathrm{E}\mathrm{k}\mathrm{e}\mathrm{d}\mathrm{k}\mathrm{i}\mathrm{n}-\mathrm{T}\mathrm{o}\mathrm{f}\mathrm{f}\mathrm{o}\mathrm{l}\mathrm{i}$-Milburn gate
In usualcomputer, we could not determine two inputs for the logical gatesAND
and OR after
we
know the output for these gates. This property is calledan
irreversibility oflogical gate. This property leads to the loss of information and
the heat generation. Thus there exists
an
upper bound ofcomputational speed.Fredkin and Toffoli proposed a conservative gate, by which any logical gate
is realized andit is showntobe
a
reversiblegate inthesense
that there isno
lossof information. This gate
was
developed by Milburnas
a quantum gate withquantum input and output. We call this gate Fredkin-Toffoli-Milburn (FTM) gate here. Recently, we reformulate a quantum channel for the FTM gate and
we rigorously study the conservation of information for FTM gate [10].
The FTM gate is composed of two input gates $\mathrm{I}_{1},$ $\mathrm{I}_{2}$ and one control gate
C. Two inputs
come
to the first beam splitter andone
spliting input passesthrough the control gate made from
an
optical Kerr device, then two splitinginputs come in the second beam splitter and appear as two outputs (Fig.2.1). Two beam splitters and the optical Kerr medium
are
needed to describe thegate.
$\mathrm{r}_{\mathrm{l}}\mathrm{g}$Z.1 $\mathrm{F}$1N1gate
(1) Beam splitters: (a) Based on [9], let $V_{1}$ be
a
mapping from $\mathcal{H}_{1}\otimes \mathcal{H}_{2}$ to $\mathcal{H}_{1}\otimes \mathcal{H}_{2}$ with transmission rate $\eta_{1}$ given by$V_{1}(|n_{1} \rangle\otimes|n_{2}\rangle)\equiv\sum_{j=0}^{n_{1}+n_{2}}C_{j}^{n_{1},n_{2}}|j\rangle\otimes|n_{1}+n_{2}-j\rangle$ (2.1)
for anyphotonnumber state vectors $|n_{1}\rangle$$\otimes|n_{2}\rangle$ $\in \mathcal{H}_{1}\otimes \mathcal{H}_{2}$. The quantumchannel
$\Pi_{BS1}^{*}$ expressing the first beam splitter (beam splitter 1) is defined by
$\Pi_{BS1}^{*}(\rho_{1}\otimes\rho_{2})\equiv V_{1}(\rho_{1}\otimes\rho_{2})V_{1}^{*}$ (2.2)
for any states $\rho_{1}\otimes\rho_{2}\in \mathrm{e}(\mathcal{H}_{1}\otimes \mathcal{H}_{2})$ . In particular, for an input state in two
gates $\mathrm{I}_{1}$ and $\mathrm{I}_{2}$ given by the tensor product of two coherent states
$\rho_{1}\otimes\rho_{2}=$
$|\theta_{1}\rangle\langle\theta_{1}|\otimes|\theta_{2}\rangle\langle\theta_{2}|,$ $\Pi_{BS1}^{*}(\rho_{1}\otimes\rho_{2})$ is written
as
$\Pi_{BS1}^{*}(\rho_{1}\otimes\rho_{2})$ $=$ $|\sqrt{\eta_{1}}\theta_{1}+\sqrt{1-\eta_{1}}\theta_{2}\rangle\langle\sqrt{\eta_{1}}\theta_{1}+\sqrt{1-\eta_{1}}\theta_{2}|$
$\otimes|-\sqrt{1-\eta_{1}}\theta_{1}+\sqrt{\eta_{1}}\theta_{2}\rangle\langle-\sqrt{1-\eta_{1}}\theta_{1}+\sqrt{\eta_{1}}\theta_{2}|$ . $(2.3)$
(b) Let $V_{2}$ be
a
mapping from $\mathcal{H}_{1}\otimes \mathcal{H}_{2}$ to $\mathcal{H}_{1}\otimes \mathcal{H}_{2}$ with transmission rate$\eta_{2}$
given by
$V_{2}(|n_{1} \rangle\otimes|n_{2}\rangle)\equiv\sum_{j=0}^{n_{1}+n_{2}}C_{j}^{n_{2},n_{1}}|n_{1}+n_{2}-j\rangle\otimes|j\rangle$ (2.4)
for anyphoton number state vectors $|n_{1}\rangle$$\otimes|n_{2}\rangle$ $\in \mathcal{H}_{1}\otimes \mathcal{H}_{2}$. Thequantumchannel
$\Pi_{BS2}^{*}$ expressing the second beam splitter (beam splitter 2) is defined by
$\Pi_{BS2}^{*}(\rho_{1}\otimes\rho_{2})\equiv V_{2}(\rho_{1}\otimes\rho_{2})V_{2}^{*}$ (2.5)
for any states $\rho_{1}\otimes\rho_{2}\in \mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{H}_{2})$. In particular, for coherent input states
$\rho_{1}\otimes\rho_{2}=|\theta_{1}\rangle\langle\theta_{1}|\otimes|\theta_{2}\rangle\langle\theta_{2}|,$ $\Pi_{BS2}^{*}(\rho_{1}\otimes\rho_{2})$ is written as
$\Pi_{BS2}^{*}(\rho_{1}\otimes\rho_{2})$ $=$ $|\sqrt{\eta_{2}}\theta_{1}-\sqrt{1-\eta_{2}}\theta_{2}\rangle\langle\sqrt{\eta_{2}}\theta_{1}-\sqrt{1-\eta_{2}}\theta_{2}|$
$\otimes|\sqrt{1-\eta_{2}}\theta_{1}+\sqrt{\eta_{2}}\theta_{2}\rangle\langle\sqrt{1-\eta_{2}}\theta_{1}+\sqrt{\eta_{2}}\theta_{2}|$ . (2.6)
(2) Optical Kerr medium: The interaction Hamiltonian in the optical Kerr medium is given by the number operators $N_{1}$ and $N_{\mathrm{c}}$ for the input system 1 and
the Kerr medium, respectively, such
as
where $\hslash$ is the Plank constant divided by $2\pi,$
$\chi$ is a constant proportional to
the susceptibility of the medium and $I_{2}$ is the identity operator
on
$\mathcal{H}_{2}$. Let $T$be the passing time of
a
beam through the Kerr medium and put $\sqrt{F}=\hslash\chi T$,a
parameter exhibiting the power ofthe Kerr effect. Then the unitary operator$U_{K}$ describing the evolution for time $\mathrm{T}$ in the Kerr medium is given by
$U_{K}=\exp(-i\sqrt{F}(N_{1}\otimes I_{2}\otimes N_{c}))$ . (2.8)
We
assume
that an initial (input) state of the control gate is the $n$ photonnumber state $\xi=.|n.\rangle\langle$$n|$, a
quantu..m
channel $\Lambda_{K}^{*}$ representing the optical Kerreffect is given by
$\Lambda_{K}^{*}(\rho_{1}\otimes\rho_{2}\otimes\xi)\equiv U_{K}(\rho_{1}\otimes\rho_{2}\otimes\xi)U_{K}^{*}$ (2.9)
for any state $\rho_{1}\otimes\rho_{2}\otimes\xi\in \mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{H}_{2}\otimes \mathcal{K})$ . In particular, for an initial state $\rho_{1}\otimes\rho_{2}\otimes\xi=|\theta_{1}\rangle\langle\theta_{1}|\otimes|\theta_{2}\rangle\langle\theta_{2}|\otimes|n\rangle\langle n|,$ $\Lambda_{K}^{*}(\rho_{1}\otimes\rho_{2}\otimes\xi)$ is denoted by
$\Lambda_{K}^{*}(\rho_{1}\otimes p_{2}\otimes\xi)$
$=$ $|\exp(-i\sqrt{F}n)\theta\rangle 1\langle\exp(-i\sqrt{F}n)\theta 1|$
$\otimes|\theta_{2}\rangle\langle\theta_{2}|\otimes|n\rangle\langle n|$, (2.10) Using the above channels, the quantum channel for the whole FTM gate is
constructed as follows: Let both
one
input and output gates be described by$\mathcal{H}_{1}$, another input and output gates be described by $\mathcal{H}_{2}$ and the control gate
be done by $\mathcal{K}$ , all of which
are
Fock spaces. Fora
total state $\rho_{1}\otimes\rho_{2}\otimes\xi$ oftwo input states and a control state, the quantum channels $\Lambda_{BS1}^{*},$$\Lambda_{BS2}^{*}$ from $\mathrm{e}(\mathcal{H}_{1}\otimes \mathcal{H}_{2}\otimes \mathcal{K})$ to $\mathfrak{S}(\mathcal{H}_{1}\otimes \mathcal{H}_{2}\otimes \mathcal{K})$ are written by
$\Lambda_{BSk}^{*}(\rho_{1}\otimes\rho_{2}\otimes\xi)=\Pi_{BSk}^{*}(\rho_{1}\otimes\rho_{2})\otimes\xi$ $(k=1,2)$ (2.11)
Therefore, the whole quantum channel $\Lambda_{\mathrm{F}\mathrm{T}\mathrm{M}}^{*}$ of the FTM gate is defined by
$\Lambda_{\mathrm{F}\mathrm{T}\mathrm{M}}^{*}\equiv\Lambda_{BS2}^{*}0\Lambda_{K}^{*}0\Lambda_{BS1}^{*}$. (2.12)
In particular, for
an
initial state $\rho_{1}\otimes\rho_{2}\otimes\xi=|\theta_{1}\rangle$ $\langle\theta_{1}|\otimes|\theta_{2}\rangle\langle\theta_{2}|\otimes|n\rangle\langle n|$,$\Lambda_{\mathrm{F}\mathrm{T}\mathrm{M}}^{*}(\rho_{1}\otimes\rho_{2}\otimes\xi)$ is obtained by
$\Lambda_{\mathrm{F}\mathrm{T}\mathrm{M}}^{*}(\rho_{1}\otimes\rho_{2}\otimes\xi)$
$=$ $|\mu_{n}\theta_{1}+\nu_{n}\theta_{2}\rangle\langle\mu_{n}\theta_{1}+\iota/_{n}\theta_{2}|$
where
.
$\mu_{k}$ $=$ $\frac{1}{2}\{\exp(-i\sqrt{F}k)+1\}$, (2.14)
$\nu_{k}$ $=$ $\frac{1}{2}\{\exp(-i\sqrt{F}k)-1\}$, $(k=0,1,2, \cdots)$ . (2.15) If $\sqrt{F}$ satisfies the conditions $\sqrt{F}=0$ or $\sqrt{F}=(2k+1)\pi(k=0,1,2, \cdots)$, then
one can obtain acomplete truth table in FTM gate.
However, it might be difficult to realize the photon number state $|n\rangle\langle$$n|$ for
the input of the Kerr medium physically. In stead of the Kerr medium, we
introduce new device related to symmetric Fock space. We contruct a quantum
logical gate mathematically with this new device in the next section.
3. Quantum logical gate
on symmetric
Fock
space
In this section,
we
reformulate beam splittings on symmetric Fock space andwe
introducea new
operator instead of the Kerr medium on that space. We discuss the mathematical formulation ofquantum logical gate bymeans
of beam splittings and the new operator.Let $G$ be a complete separable metric space and $\mathcal{G}$ be a Borel $\sigma$-algebra of
G. $v$ is called a locally finite diffuse
measure
on the measurable space $(G, \mathcal{G})$ if$v$ satisfies the conditions (1) $v(K)<\infty$ for bounded $K\in \mathcal{G}$ and (2) $v(\{x\})=0$
for any $x\in G$. We denote the set of all finite integer- valued measures $\varphi$ on
$(G, \mathcal{G})$ by $M$. For a set $K\in \mathcal{G}$ and
a
nutural number $n\in \mathbb{N}$,we
put the set of$\varphi$ satisfying $\varphi(K)=n$ as
$M_{K,n}\equiv\{\varphi\in M;\varphi(K)=n\}$ .
Let $\mathfrak{M}$ be
a
$\sigma$-algebra generated by $M_{K,n}$. $F$ is the $\sigma$-finitemeasure on
$(G, \mathcal{G})$defined by
$F(Y) \equiv 1_{Y}(\varphi_{0})+\sum_{n=1}\frac{1}{n!}\int_{G^{n}}1_{Y}(\sum_{j=1}^{n}\delta_{x_{j}})v^{n}(dx_{1}\cdots dx_{n})$,
where $1_{Y}$ is the characteristic function of
a
set $Y,$ $\varphi_{0}$ isan
empty configulationFock space. We define an exponetal vector $\exp_{g}$ : $Marrow \mathbb{C}$ generated by
a
givenfunction $g:Garrow \mathbb{C}$ such that
$\exp_{g}(\varphi)\equiv\{$
1 $(\varphi=\varphi_{0})$ ,
$\prod_{x\in\varphi}g(x)$ $(\varphi\neq\varphi_{0})$,
$(\varphi\in M)$ .
3.1. Generalized beam splittings on Fock space
Let $\alpha,$$\beta$ be measurable mappings from $G$ to
$\mathbb{C}$ satisfying $\overline{\alpha}$
$|\alpha(x)|^{2}+|\beta(x)|^{2}=1$, $x\in G$.
We intoduce
an
unitary operator $V_{\alpha,\beta}$:
$\mathcal{M}\otimes \mathcal{M}arrow \mathcal{M}\otimes \mathcal{M}$ defined$\mathrm{b}$
$(V_{\alpha,\beta}\Phi)(\varphi_{1}, \varphi_{2})$ $\equiv$
$\sum_{\hat{\varphi}_{1}\leq\varphi_{1}}\sum_{\hat{\varphi}_{2}\leq\varphi_{2}}\exp_{\alpha}(\hat{\varphi}_{1})\exp_{\beta}(\varphi_{1}-\hat{\varphi}_{1})$
$\cross\exp_{-\overline{\beta}}(\hat{\varphi}_{2})\exp_{\overline{\alpha}}(\varphi_{2}-\hat{\varphi}_{2})$
$\cross\Phi(\hat{\varphi}_{1}+\hat{\varphi}_{2}, \varphi_{1}+\varphi_{2}-\hat{\varphi}_{1}-\hat{\varphi}_{2})$
for $\Phi\in \mathcal{M}\otimes \mathcal{M}$ and $\varphi_{1},$$\varphi_{2}\in M$. Let $A\equiv \mathrm{B}(\mathcal{H})$ be the set of all bounded
opera-tors
on
$\mathcal{M}$ and 6$(A)$ be the set of all normal stateson
A.
$\mathcal{E}_{\alpha,\beta}$ : $A\otimes Aarrow A\otimes A$defined by
$\mathcal{E}_{\alpha,\beta}(C)\equiv V_{\alpha}^{*},{}_{\beta}CV_{\alpha,\beta}$, $\forall C\in A\otimes A$
is the lifting in the
sense
of Accardi and Ohya [1] and the dual map $\mathcal{E}_{\alpha,\beta}^{*}$ of$\mathcal{E}_{\alpha,\beta}$given by
$\mathcal{E}_{\alpha,\beta}^{*}(\omega)(\bullet)\equiv\omega(\mathcal{E}_{\alpha,\beta}(\bullet))$, $\forall\omega\in \mathfrak{S}(A\otimes A)$
is the CP channel from $\mathfrak{S}(A\otimes A)$ to $\mathfrak{S}(A\otimes A)$ . Using the exponetial vectors,
one can
denote a coherent state $\theta^{f}$ Bby$\theta^{f}(A)\equiv\langle$
$\exp_{f},$ A$\exp_{f}\rangle$
$e^{-||f||^{2}}$
, $\forall f\in L^{2}(G, \iota/),$ $\forall A\in A$.
In particular, for the input coherent states $\eta_{0}\otimes\omega_{0}=\theta^{f}\otimes\theta^{g}$, two output states
$\omega_{1}(\bullet)\equiv\eta_{0}\otimes\omega_{0}(\mathcal{E}_{\alpha,\beta}((\bullet)\otimes I))$and $\eta_{1}(\bullet)\equiv\eta_{0}\otimes\omega_{0}(\mathcal{E}_{\alpha,\beta}(I\otimes(\bullet)))$ are obtained
by $\omega_{1}$ $=$ $\theta^{\alpha f+\beta g}$ , $\eta_{1}$ $=$ $\theta^{-\overline{\beta}f+\overline{\alpha}g}$ .
$\mathcal{E}_{\alpha,\beta}^{*}$ is called
a
generalized beamsplittingon
Fock space because it also hold thesame
properties satisfied by the generated beam splitting $\Pi^{*}\mathrm{i}\mathrm{n}$ Section 1.Now we introduce a self-adjoint unitary operator $\tilde{U}$
, which denotes a new
device instead ofthe Kerr medium, defined by
$\tilde{U}(\Phi)(\varphi_{1}, \varphi_{2})\equiv(-1)^{|\varphi_{1}||\varphi_{2}|}\Phi(\varphi_{1}, \varphi_{2})$
for $\Phi\in \mathcal{M}\otimes \mathcal{M}$ and
$\varphi_{1},$$\varphi_{2}\in G$, where $|\varphi_{k}|\equiv\varphi_{k}(G)$ $(k=1,2)$. For the input
state $\omega_{1}\otimes\kappa\equiv\theta^{f}\otimes\frac{1}{||\psi||^{2}}\langle\psi, \bullet\psi\rangle$, the output state $\omega_{2}$ of new device is
$\omega_{2}(A)$ $\equiv$ $\omega_{1}\otimes\kappa(\tilde{U}(A\otimes I)\tilde{U})$
$=$ $\frac{1}{||\psi||^{2}}\int_{M}F(d\varphi)|\psi(\varphi)|^{2}\theta^{(-1)^{|\varphi|^{2}f}}(A)$
for any $A\in A,$ $\psi\in \mathcal{M}(\psi\neq 0)$ and $f\in L^{2}(G, \nu)$
.
If $\kappa$ is given by thevacuum
state $\theta^{0}$
, then the output state$\omega_{2}$ is equals to$\omega_{1}$ and if$\kappa$is given by oneparticle
state, that is, $\kappa=\frac{1}{||\psi||^{2}}\langle\psi, \bullet\psi\rangle$ with $\psi[_{M_{1}^{c}}$(where $M_{1}$ is the set of one-particle
states), then $\omega_{2}$ is obtained by
$\theta^{-f}$.
Let $M_{o}$ (resp. $M_{e}$) be the set of $\varphi\in M$
which satisfies that $|\varphi|$ is odd (resp. even) and $M$ be the union of$M_{o}$ and
$M_{e}$.
The output states $\omega_{2}$ of the
new
device is written by$\omega_{2}(A)=\lambda_{1}\theta^{-f}(A)+\lambda_{2}\theta^{f}(A)$ $\forall A\in A$,
where $\lambda_{1}$ and $\lambda_{2}$ are given by
$\{$
$\lambda_{1}=\frac{1}{||\psi||^{2}}\int_{M_{o}}F(d\varphi)|\psi(\varphi)|^{2}$ , $\lambda_{2}=\frac{1}{||\psi||^{2}}\int_{M_{e}}F(d\varphi)|\psi(\varphi)|^{2}$
Two output states$\omega_{3}(\bullet)\equiv\omega_{2}\otimes\eta_{2}(\mathcal{E}_{\alpha_{2},\beta_{2}}((\bullet)\otimes I))$ and$\eta_{3}(\bullet)\equiv\omega_{2}\otimes\eta_{2}(\mathcal{E}_{\alpha_{2},\beta_{2}}(I\otimes(\bullet)))$
ofthe total logical gate including two beam splittings$\mathcal{E}_{\alpha_{k},\beta_{k}}^{*}$ with $(|\alpha_{k}|^{2}+|\beta_{k}|^{2}=1)$
$(k=1.2)$ and the
new
device instead ofKerr medium are obtained by$\omega_{3}$ $=$ $\lambda_{1}\theta^{\alpha_{2}(-(\alpha_{1}f+\beta_{1}g))+\beta_{2}(-\overline{\beta}_{1}f+\overline{\alpha}_{1g})_{+\lambda_{2}\theta^{\alpha_{2}(\alpha_{1}f+\beta_{1}g)+\beta_{2}(-\beta_{1}f+\overline{\alpha}_{1\mathit{9}})}}}$ , $\eta_{3}$ $=$ $\lambda_{1}\theta^{-\beta_{2}(-(\alpha_{1}f+\beta_{1}g))+\overline{\alpha}_{2}(-\overline{\beta}_{1}f+\overline{\alpha}_{1\mathit{9}})_{+\lambda_{2}\theta^{-\overline{\beta}_{2}(\alpha_{1}f+\beta_{1}g)+\overline{\alpha}_{2}(-\overline{\beta}_{1}f+\overline{\alpha}_{1\mathit{9}}}}})$ , where $\omega_{2}=\lambda_{1}\theta^{-(\alpha_{1}f+\beta_{1}g)}+\lambda_{2}\theta^{\alpha_{1}f+\beta_{1}g}$and $\eta_{2}=\eta_{1}=\theta^{-\overline{\beta}_{1}f+\overline{\alpha}_{1\mathit{9}}}$.
3.2. Complete truth table for the
new
logical gateIn this section,
we
show the complete truth table giving by the above logicalgate on Fock space.
We put $\omega_{1}=\theta^{f}$ and $\eta_{1}=\theta^{g}$. Ifwe assume the case (1) of $\lambda_{1}=0$ and $\lambda_{2}=1$,
then
one
has$\omega_{3}$ $=$ $\theta^{(\alpha_{1}\alpha_{2}-\beta_{1}\beta_{2})f+(\alpha_{2}\beta_{1}+\overline{\alpha}_{1}\beta_{2})g}$ , $\eta_{3}$ $=$ $\theta^{(-\alpha_{1}\overline{\beta}_{2}-\overline{\alpha}_{2}\beta_{1})f+(-\beta_{1}\beta_{2}+\overline{\alpha}_{1}\overline{\alpha}_{2})_{\mathit{9}}}$ ,
and ifwe
assume
the case (2) of $\lambda_{1}=1$ and $\lambda_{2}=0$, then one has$\omega_{3}$ $=$ $\theta^{(-\alpha_{1}\alpha_{2}-\overline{\beta}_{1}\beta_{2})f+(\alpha_{2}\beta_{1}+\overline{\alpha}_{1}\beta_{2})g}$ , $\eta_{3}$ $=$ $\theta(\alpha_{1}\overline{\beta}_{2}-\overline{\alpha}_{2}\overline{\beta}_{1})f+(-\beta_{1}\overline{\beta}_{2}+\overline{\alpha}_{1}\overline{\alpha}_{2})g$ .
For example, we have the complete truth tables for the following two
cases
(I) and (II): (I) When $\alpha_{1}=\alpha_{2}=\beta_{1}=\beta 2=\frac{1}{\sqrt{2}}$are
satisfied, two output statesof the new logical gate become $\omega_{3}=\theta^{g}$ and $\eta_{3}=\theta^{-f}$ under the
case
(1) and $\omega_{3}=\theta^{-f}$ and $\eta_{3}=\theta^{g}$ under thecase
(2). (II) When $\alpha_{k}=\frac{e^{i\gamma_{k}}}{\sqrt{2}}$ and $\beta_{k}=\frac{e^{i\delta_{k}}}{\sqrt{2}}$with $\gamma_{k},$ $\delta_{k}\in[0,2\pi]$ hold
$\alpha_{1}\alpha_{2}=\beta_{1}\overline{\beta}_{2}$,
one
has $\gamma_{1}+\gamma_{2}=\delta_{2}-\delta_{1}$ and two outputstates of the new logical gate become $\omega_{3}=\theta^{g}$ and $\eta_{3}=\theta^{-f}$ under the case (1)
and $\omega_{3}=\theta^{-f}$ and $\eta_{3}=\theta^{g}$ under the
case
(2).The new logical gate treats three initial states $\omega_{0},$$\eta_{0}$ and $\kappa$ corresponding to
two input gates $I_{1},$$I_{2}$ and the control gate $C$, respectively. The true $T$ and false
$F$ of the input state $\omega$ (resp. $\eta$) are described by two different states
$\omega^{T}$ and $\omega^{F}$ (resp. $\eta^{T}$ and $\eta^{F}$), that is;
Hue $\Leftrightarrow$ coherent state
$\omega^{T}=\theta^{f}$ (resp. $\eta^{T}=\theta^{g}$),
False $\Leftrightarrow$
vacuum
state.$\omega^{F}=\theta^{0}$ (resp. $\eta^{F}=\theta^{0}$).
Moreover, the truth state $\kappa^{T}$ and the false state $\kappa^{F}$
are
denoted by ffie controlstates of the
case
(1) and (2), respectively. When the initial control state $\kappa$is $\kappa^{F}$ under the above case (I) or (II), the final states of the new logical gate
corresponding to two input gates $O_{1},$$O_{2}$ are obtained as the following truth
(3.1)
When the initial control state $\kappa$ is
$\kappa^{\mathit{1}}$ under the above
case
(I)or
(II), thefinal states ofthe new logical gate corresponding to two input gates $O_{1},$ $O_{2}$ are
obtained
as
the following truth table:(3.2)
It means that the new logical
gaee
perrormsrne
complete truth table. Furtherresults will be appear in
our
joint paper [11].References
[1] L.Accardi and M.Ohya, Compound channels, transition expectations, and
liftings, Appl. Math. Optim. Vol.39, 33-59,
1999.
[2] K.H. Fichtner, W. Freudenbergand V. Liebscher, Beam splittings and time evolutions of Boson systems, Fakult\"at f\"ur MathematikB und Informatik, $\mathrm{M}\mathrm{a}\mathrm{t}\mathrm{h}/\mathrm{I}\mathrm{n}\mathrm{f}/96/39$, Jena, 105, 1996.
[3] E.Fredkin and T. Toffoli, Conservative logic, International Journal of The-oretical Physics, 21, pp.
219-2531982.
[4] G.J. Milburn, Quantumoptical Fredkin gate, Physical Review Letters, 62,
2124-2127, 1989.
[5] M.Ohya, Quantum ergodic channels in operator algebras, J. Math. Anal.
Appl. 84, pp. 318-327, 1981.
[6] M.Ohya, On compound state and mutual information in quantum informa-tion theory, IEEE Trans. Information Theory, 29, pp. 770-777, 1983.
[7] M. Ohya, Some aspects of quantum information theory and their applica-tions to irreversible processes, Rep. Math. Phys., 27, pp. 19–47,
1989.
[8] M. Ohya and D. Petz, Quantum Entropy and Its Use, Springer, 1993. [9] M. Ohya and N. Watanabe, Construction and analysis of a mathematical
model in quantum communication processes, Electronics and
Communica-tions in Japan, Part 1, Vol.68, No.2, 29-34, 1985.
[10] M. Ohyaand N. Watanabe, On
mathematical
treatment ofoptical Fredkin-Toffoli-Milburn
gate, Physica D, Vo1.120, 206-213,1998.
[11] W. Freudenberg, M. Ohya and N. Watanabe,